

Next: Solid cylinder transient 1-D.
Up: Cylindrical Coordinates. Transient 1-D.
Previous: Infinite body, cylindrical coordinate,
R10 Infinite region with circular hole,
a < r < 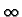 ,
with G = 0 (Dirichlet) at r = a.
,
with G = 0 (Dirichlet) at r = a.
GR10(r, t 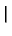 r r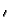 , ,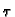
 ) ) |
= |
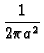 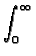 exp exp -
- 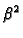 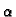 (t - (t - 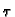 )/a2 )/a2![$\displaystyle \left.\vphantom{ -\beta ^{2}\alpha (t-\tau )/a^{2}}\right]$](img275.gif) ![$\displaystyle {\frac{%
\beta \,R(r)\,R(r^{\prime })}{\left[ J_{0}(\beta )\,\right] ^{2}+\left[
Y_{0}(\beta )\,\right] ^{2}}}$](img276.gif) d d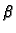 |
|
| where R(r) |
= |
J0(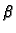 r/a)Y0( r/a)Y0(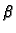 ) - Y0( ) - Y0(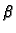 r/a)J0( r/a)J0(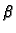 ) ) |
|
R20 Infinite region with circular hole,
a < r < 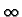 ,
with
,
with
 G
G r = 0 (Neumann) at r = a.
r = 0 (Neumann) at r = a.
GR10(r, t 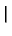 r r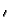 , ,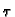
 ) ) |
= |
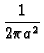 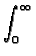 exp exp -
- 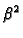 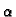 (t - (t - 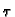 )/a2 )/a2![$\displaystyle \left.\vphantom{ -\beta ^{2}\alpha (t-\tau )/a^{2}}\right]$](img275.gif) ![$\displaystyle {\frac{%
\beta \,R(r)\,R(r^{\prime })}{\left[ J_{1}(\beta )\,\right] ^{2}+\left[
Y_{1}(\beta )\,\right] ^{2}}}$](img277.gif) d d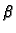 |
|
| where R(r) |
= |
J0(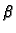 r/a)Y1( r/a)Y1(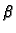 ) - Y0( ) - Y0(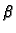 r/a)J1( r/a)J1(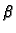 ) ) |
|
Note that
J0(z)Y1(z) - Y0(z)J1(z) = - 2/(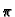 z).
z).
R30 Infinite region with circular hole,
a < r < 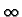 ,
with
- k
,
with
- k G
G r + hG = 0 (convection) at r = a.
r + hG = 0 (convection) at r = a.
GR10(r, t 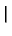 r r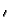 , ,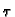
 ) ) |
= |
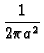 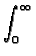 exp exp -
- 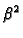 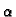 (t - (t - 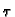 )/a2 )/a2![$\displaystyle \left.\vphantom{ -\beta ^{2}\alpha (t-\tau )/a^{2}}\right]$](img275.gif) 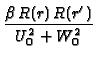 d d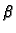 |
|
| where R(r) |
= |
W0J0(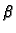 r/a) - U0Y0( r/a) - U0Y0(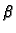 r/a) r/a) |
|
| W0 |
= |
- 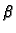 Y1( Y1(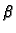 ) - BY0( ) - BY0(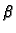 ) ) |
|
| U0 |
= |
- 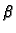 J1( J1(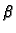 ) - BJ0( ) - BJ0(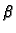 ) ) |
|
| and B |
= |
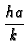 |
|


Next: Solid cylinder transient 1-D.
Up: Cylindrical Coordinates. Transient 1-D.
Previous: Infinite body, cylindrical coordinate,
Kevin D. Cole
2002-12-31
![$\displaystyle {\frac{%
\beta \,R(r)\,R(r^{\prime })}{\left[ J_{0}(\beta )\,\right] ^{2}+\left[
Y_{0}(\beta )\,\right] ^{2}}}$](img276.gif) d
d![$\displaystyle {\frac{%
\beta \,R(r)\,R(r^{\prime })}{\left[ J_{0}(\beta )\,\right] ^{2}+\left[
Y_{0}(\beta )\,\right] ^{2}}}$](img276.gif) d
d![]() ,
with
,
with
![]() G
G![]() r = 0 (Neumann) at r = a.
r = 0 (Neumann) at r = a.
![$\displaystyle {\frac{%
\beta \,R(r)\,R(r^{\prime })}{\left[ J_{1}(\beta )\,\right] ^{2}+\left[
Y_{1}(\beta )\,\right] ^{2}}}$](img277.gif) d
d![]() ,
with
- k
,
with
- k![]() G
G![]() r + hG = 0 (convection) at r = a.
r + hG = 0 (convection) at r = a.
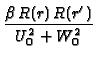 d
d